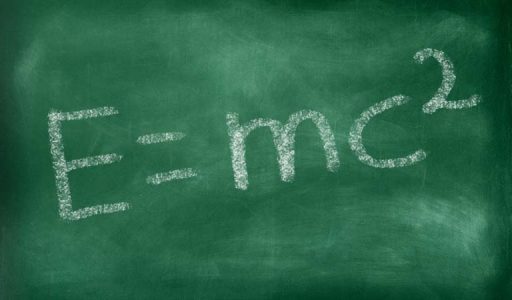Acceleration in special relativity is not parallel to the applied force. This is in contrast to the case of classical mechanics where Newton’s second law implies that force and acceleration are parallel by definition. In the present article, this result is derived in a simple form.
In the present article, we consider the collision of two particles \(A\), and \(B\), with \(B\) at rest in the lab frame of reference before the collision. As a result of the collision one or more particles \(C,D,\dots\), are produced. The produced particles can be, in principle, different from the colliding ones.
In the present article, the Lorentz transformations of the space-time coordinates, velocities, energy, momentum, accelerations, and forces, are presented in a condensed form. It is explained how the Lorentz transformation for a boost in an arbitrary direction is obtained, and the relation between boosts in arbitrary directions and spatial rotations is discussed.
The equation \(E=Mc^2\) is probably one of the most famous equations in the history of the physics, and its meaning has been amply discussed. However, it is generally unknown how Einstein got this beautiful result. In the present post I will show you a derivation of this equation.